Even if your crew has taken all the necessary precautions in paying out cable and handling the reels, a cable pull can still go sour if you damage the cable's outer insulation during the process. However, with the help of a few calculations and a working knowledge of arithmetic, you can prevent problems in recently energized feeders by calculating the maximum allowable pulling tension for any installation — and you don't even need to know calculus.
Mathematical proficiency aside, you do have to know the following installation parameters:
- Raceway size
- Cable configuration
- Correction factor for cable weight
- Jamming potential
- Conductor clearance
- Sidewall bearing pressure
Now, let's see how these factors apply in a sample pulling tension calculation.
Sample power feeder installation
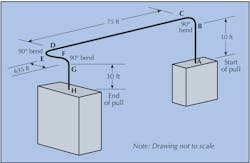
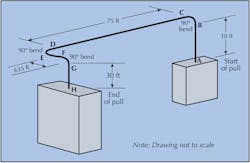
Suppose you're involved in a design/build project for a paper mill and your client needs a 400A, 15kV feeder to run as shown in Fig. 1.The client has required all feeders on the site to be pull-in-pull-out type in galvanized rigid steel (GRS) conduit. The client has also stipulated you use 90°C, single-conductor, grounded neutral, MV cables with tape shielding; XLPE insulation; and an overall PVC jacket. After referring to NEC Table 310.73, size the feeder at 500kcmil. With these requirements in hand, check with the cable manufacturer and you'll find the MV cable you need has an outer diameter (d) of 1.60 in. and a weight of 2.2 lb/ft.
Now it's time to size the conduit. Table 1 in Chapter 9 of the NEC, states the allowable percentage of conductor fill is 40%. You can calculate the total area of the three MV cables using the following equation:
Area = 3 x (pi ÷ 4) x d2
Area = 3 x .785 x 1.602
Area = 6.03 sq. in.
In this situation, Table 4 (Rigid Metal Conduit) in Chapter 9 of the NEC calls for a 5-in. conduit. This conduit size will allow you to slip under the allowable percentage of conductor fill by 10%.
Position makes a difference
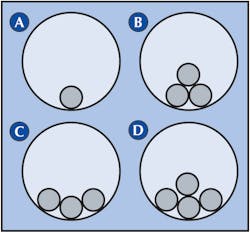
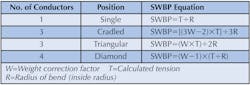
It may not seem important, but each cable's geometric position (Fig. 2)) has a unique effect on the amount of frictional force, or drag, the conductors endure during the pull. In addition, the positioning affects the weight factor. By using the ratio of the raceway's inside diameter (D) to the conductor's outside diameter (d), you can find what geometric position you can expect to see.
Although the position of one cable is easy to predict (see option A in Fig. 2), other positions are not as obvious:
- Triangular (option B in Fig. 2): This occurs when you pull three individual conductors from three separate reels, and their D/d ratio is less than 2.5. If you pull individual triplexed conductors from a single reel, they also will sit in this position.
- Cradle (option C in Fig. 2): This position may occur when you pull three individual conductors from three separate reels, and their D/d ratio is between 2.5 and 3.0. This position is the least favorable because it yields the worst-case scenario of drag during the pull.
- Diamond (option D in Fig. 2): This position occurs when you pull four individual conductors from four separate reels, and their D/d ratio is less than 3.0. If you pull quadruplexed individual conductors from a single reel, the multiconductor cable will also sit in this position.
To determine how the conductors will sit in the conduit, refer to Table 4 for the inside diameter (D) of the 5-in. GRS conduit, which is 5.07 in. Use the ratio of the conduit's inside diameter (D) to the cable's outside diameter (d) to find how the single conductors will sit in the conduit. In this case, this ratio is:
D ÷ d
5.07in. ÷ 1.60 in.
= 3.17
Because this ratio results in a number greater than 3.0, the individual conductors will sit in a cradle configuration in the conduit.
Conductors “weigh” more than you think
Now that you know the cable positioning, it's necessary to determine how the conductors' weight will affect the pull.
Weight correction factor is important because when you pull two or more conductors in a raceway, the sum of the forces developed between the conductors and the raceway is always greater than the sum of the individual conductor weights.
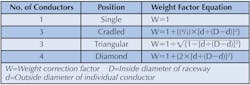
The equations in Table 1 for determining weight correction factor for specific installations are based on the inside diameter of the raceway and the outside diameter of the conductor.When you have three single conductors of equal diameter and weight (which is the most common scenario), you can expect a higher weight factor for the cradle position than the triangular position. What does this mean to you? It means you should assume the conductors would sit in the cradle position (unless you are pulling triplexed individual conductors from a single reel), because this will yield a higher and more conservative pulling tension calculation. Use the following equation to find the weight correction factor:
W = 1 + {(4 ÷ 3) x [d ÷ (D-d)2}
W = 1 + {(4 ÷ 3) x [160 ÷ (3.47)2}
W = 1.28
Don't jam those cables
When sizing your raceway system, you should always consider the possibility of cables jamming or wedging. This usually occurs when you have three or more individual conductors lying side by side in a single plane. As you pull the conductors through a bend, the curvature of the bend tends to squeeze the conductors together.
However, if you're pulling one or two conductor cables, a multiconductor cable with an overall jacket, or a nonjacketed multiconductor cable made of a triplexed or quadruplexed conductor assembly, you probably don't have to worry about jamming.
Use the following formula to determine the likelihood of jamming. Use the inside diameter of the raceway and the outside diameter of the individual conductor:
1.05 x (D ÷ d)
The constant 1.05 factor is a reflection of the fact that bends are actually oval in the sectional view.
- If the value is less than 2.5, you will not have a jamming problem.
- If the value is less than 3.0 but greater than 2.8, jamming is very possible.
- If the value is greater than 3.0, you won't have a jamming problem.
Note: Keep away from jam ratios of 2.8 to 3.2 for Type MV extruded dielectric power cables.
Using the values for inside diameter of the raceway and the outside diameter of the individual conductor from the example, you get the following value:
1.05 x (D ÷ d)
1.05 x (5.07 in. ÷ 1.60 in.)
= 3.33
Because this calculation results in a number greater than 3.0, you probably won't have a jamming problem.
Conductors need headroom, too
Don't forget you must also have adequate clearance between the uppermost conductor and the top of the raceway to ensure a safe and easy pull. For straight pulls, you can have a clearance as small as ¼ in. and still be safe. For more complex pulls, you should have between ½ in. and 1 in.
Use the equations in Table 2 (which are based on worst-case scenarios) to find the clearance distance for a given raceway and cable sitting position.Note that these equations include a 5% increase (a factor 1.05) to compensate for variations in cable and raceway diameters and the oval shape of the raceway sections at bends. However, because the conductors in the current example will sit in the cradle position, you will not need to check for clearance.
Calculating the pulling tension
Now that you've checked most of the factors that affect cable pulling, it's time to start calculating the pulling tension, using the following equation:
T = L x w x f x W
where T is the total pulling tension (lb), L is the length (ft) of cable feeder you're pulling, w is the total weight (lb/ft) of the conductors, f is the coefficient of friction (usually 0.5 for well-lubricated conditions), and W is the weight correction factor. (See Table 3 for coefficients of friction of various raceway/cable configurations.)
Step 1: TA-B = 10 ft x 6.6 lb/ft x 0.5 x 1.28
TA-B = 42 lb
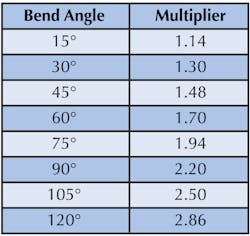
Step 2: TA-C = TA-B 90° bend multiplier
TA-C = 42 lb x 2.2
TA-C = 92 lb
Step 3: TC-D = 75 ft x 6.6 lb/ft x 0.5 x 1.28
TC-D = 317 lb
Step 4: TA-D = TA-C + TC-D
TA-D = 92 lb + 317 lb
TA-D = 409 lb
Step 5: TA-E = TA-D x 90° bend multiplier
TA-E = 409 lb x 2.2
TA-E = 900 lb
Step 6: TE-F = 635 ft x 6.6 lb/ft x 0.5 x 1.28
TE-F = 2,682 lb
Step 7: TA-F = TA-E + TE-F
TA-F = 900 lb + 2,682 lb
TA-F = 3,582 lb
Step 8: TA-G = TA-F x 90° bend multiplier
TA-G = 3,582 lb x 2.2
TA-G = 7,880 lb
Step 9: TG-H = 30 ft x 6.6 lb/ft x 0.5 x 1.28
TG-H = 127 lb
Step 10: TA-H = 7,880 lb + 127 lb
TA-H = 8,007 lb
Based on the proper calculations, you'll need approximately 8,000 lb of pulling tension to pull the 15kV conductors, but you're not done yet.
Cables are sensitive to pressure on their walls
The final step in the cable-pulling process is determining whether the pulling tension will compromise the conductor's sidewall bearing pressure limit. When you pull a cable or individual conductors through a raceway bend or around a sheave, sidewall bearing pressure (SWBP) develops between the cable or conductor wall and the bend or sheave.
This pressure has a very dramatic effect on power feeder raceway system design because it relates directly to the radii of bends, pulling tension, and the cable or conductors' weight. Most of the time, you can omit this weight factor from your SWBP calculation because it's relatively small in comparison to pulling tension.
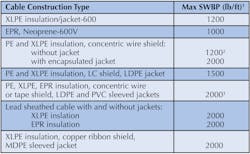
Usually, SWBP is expressed in terms of tension out of the bend (lb), divided by the bend radius (ft). The calculated result is a unit of force-per-unit-length. Use the equations in Table 5 to find the SWBP for various cable/raceway configurations and specific radius bends.
- Cradle position: center conductor.
- Diamond position: bottommost conductor.
- Triangular position: bottom two conductors.
You can use these limits in your raceway system design efforts. For example, if the design calls for pulling three single-conductor 600V XLPE conductors around a bend and your pulling tension calculation yields a value of 3,600 lbs, then the minimum bend radius would be 3,600 lbs divided by 1,200 lbs/ft, or 3 ft. Verify the three 90° bends have a sufficient radius to limit the SWBP on the conductors to 750 lb.
Because tension TA-C (92 lb) is relatively small, you can use standard elbows and not worry about exceeding the 750-lb SWBP limit. Tension TA-G, however, is another matter — it is imperative that you don't exceed the 750-lb SWBP limit.
Use the SWBP equation for a cradled position and solve for radius (R):
SWBP = [(3W - 2) x T] ÷ 3R
750 = {[(3 x 1.28) - 2] x 7,880} ÷ 3R
R = 14,499 ÷ 2,250 = 6.44 ft
This means you need to bend a 10-ft length of conduit into a large-radius sweep. (You'll need the extra length to compensate for the bend.)
Pulling cable is hard enough if you know what you're doing, so failing to follow the proper procedure can make the job that much more arduous, not to mention pointless if your feeders fail shortly after the pull. Because even the slightest oversights in determining maximum allowable pulling tension can cause problems in new feeders, it's crucial that you implement the correct calculations to get the job done right the first time.