Power Factor, Capacitors, Harmonic Filters, and Resonance — Part 1
According to the “IEEE Standard Dictionary of Electrical and Electronic Terms,” power factor (PF) is defined as “the ratio of the circuit power (watts) to the circuit volt-amperes.” This is described by the equation PF = W ÷ VA. Put another way, PF is the ratio of useful power to perform real work (commonly termed “active power”) to the power supplied by an electric utility (commonly termed “apparent power”). Usually, the goal is to get the PF of a facility to about 0.90 or higher.
Low PF (below 0.90) occurs when the volt-ampere drawn by a load exceeds the wattage used by the load. From an electric utility perspective, low PF is primarily caused by inductive loads and overhead, pole-mounted electrical distribution systems. Improving PF by adding shunt capacitance to power distribution networks has been and remains a common electric utility practice traceable back to the earliest uses of alternating current. Large industrial and commercial firms have also used capacitors to add offsetting reactive currents to balance out reactive currents from inductive loads within the facility and thereby improve PF.
While electric utilities use capacitors for voltage support and to maintain distribution capacity, the use of capacitor networks by end-users is usually driven by electricity rates or the need to free up distribution capacity. The practice of adding offsetting reactive loads (capacitive or inductive) falls into a general category that we can conveniently call displacement PF.
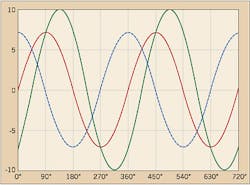
There is a time difference or phase angle between voltage and current waveforms, and cos U reflects the PF caused by the phase angle. For instance, a 45° displacement equates to a 0.707 displacement PF. Figure 1 shows PF correction of sinusoidal, displaced waveforms. The green trace is a 45° lagging waveform from an inductive load; the blue dashed line is a 90° offsetting waveform from shunt capacitance; and the red trace is the corrected in-phase waveform. The amplitude of the corrected waveform (red) is smaller than the original waveform (green) because the inductive components are balanced out. You can calculate the offsetting current by using the following equation:
Icorrection = sin U × Idisplacement
In this case, sin U = 0.707, so the offsetting current is 7.07 (10 × 0.707).
Armed with all of this PF knowledge, nothing can go wrong, right? Wrong! All sorts of mischief can arise, leaving you confused and searching for answers. At best, the problems can be embarrassing; at worst, they can be catastrophic. At the root of these problems are the unforeseen effects of resonance, and both simple capacitor banks and complex harmonic filters can be adversely affected. Source inductance (normally transformer leakage inductance) can interact with harmonic currents and cause unwanted voltage distortion. Worse yet, source inductance and shunt capacitance can interact, forming resonant tank circuits (Fig. 2).
The short form equation for resonance is as follows:
hr = 1 ÷ (2ϖ√LC) (Equation 1)
This equation clearly shows that the two major players are inductance (L) and capacitance (C). If the resonant circuits occur at integer multiples of the fundamental frequency, then all sorts of mayhem can occur, such as fuses blowing, breakers tripping, capacitors blowing up, and loads tripping off-line.
Let's see how the effects of resonance can affect a single stage capacitor bank.
Resonance case study
Our case history involves a facility where voltage distortion increased markedly, following nonlinear load concentrations, at various times throughout the day. Figure 3 shows service bus voltage without nonlinear loads operating while Fig. 4 shows service bus voltage with nonlinear loads operating. As you can see, voltage distortion increased from below 2%THD (without nonlinear loads operating) to 6.9%THD (with nonlinear loads operating). Dominant frequencies and distortion levels (%fundamental) for Fig. 4 were the 11th order harmonic at 6.1% and the 13th order harmonic at 2.3%.
When the voltage distortion rose sufficiently inside the facility, variable-speed drives would turn off and disrupt operations. Also, fuses would blow causing total disruption, and capacitors occasionally failed catastrophically.
Looking at the current flowing through the capacitor bank, the effects of the harmonic currents are even more evident. Figure 5 shows current flowing through the bank with and without nonlinear loads operating. With nonlinear loads operating, the 13th harmonic currents were dominant (44.6% of the 269A fundamental or 120A). The harmonic currents added to the fundamental root-mean-squared (rms) level of 60 Hz current. Without harmonic loads operating, the rms current was 236A; with harmonic loads operating, the rms current increased to 311A. At some point, fuses gave way but this was only part of the problem. Figure 6 shows the squared values of the currents shown in Fig. 5.
Remember, I2R losses involve resistance and squared current. The highest squared value with harmonic currents present is 2.48 times larger than the highest squared value without harmonic currents. This amounts to substantial instantaneous heating differences, resulting in blown fuses and capacitors — not exactly what was intended when the capacitors were installed.
Harmonic and reactive currents
With the advent of harmonic currents from nonlinear loads, users have learned that low PF is also caused by harmonic currents. However, they may not have totally embraced the negative consequences caused by mistaking the content. The equation for true PF (PF = W 4 VA) is not limited solely to displacement effects. It also addresses both the effects of harmonic currents and displacement at the fundamentals. However, users must recognize that measured values for PF contain both fundamental displacement and harmonic components, along with harmonic content. Ignore the harmonic content and problems will occur.
The following IEEE standards provide recommendation guides you can use to either offset reactive currents or to address harmonic currents:
-
IEEE Std. 18-2002, “IEEE Standard for Shunt Power Capacitors”
-
IEEE Std. 1036-1992, “IEEE Guide for Application of Shunt Power Capacitors”
-
IEEE Std. 519-1992, “IEEE Recommended Practices and Requirements for Harmonic Control in Electrical Power Systems.”
Continue on Page 2
IEEE 519 provides a way to estimate harmonic resonance for a facility before capacitors are installed. You can use the following equations to estimate harmonic frequency:
hr = √(MVAsc 4 Mvarcap) = √(Xc 4Xsc)
Where,
hr = Resonant frequency as a multiple of the fundamental frequency
MVAsc = Short circuit value for the service
Mvarcap = Capacitor rating at the system voltage
Xc = Capacitive reactance of the capacitor bank at the fundamental frequency
Xsc = Short-circuit reactance at the substation.
The main determinant of short-circuit current for a facility is the inductance of the electric utility transformer serving it. The electric utility may be able to provide you with the transformer inductance as well as the short circuit current. If this is the case, then calculating potential resonance is much easier. However, if the transformer inductance is not available, then you can approximate the leakage inductance by using the transformer impedance along with the rated voltage and current values:
Find the full load capacity: Transformer VA ÷ 3 = single-phase VA
Find the single-phase circuit capacity (A): Single-phase VA ÷ L-N voltage rating
Find short circuit capacity (base impedance in ohms): Single-phase current divided by rated transformer impedance
Find the inductance (L): base impedance (ohms) ÷ (2 × ϖ × 60)
If the above estimation processes indicate that the use of a capacitor bank poses a resonance risk, you can tune a simple bank to a nonharmonic frequency by adding series inductance (Fig. 7).
In reality, the aforementioned estimation processes are the basic building blocks for many passive harmonic filters. As noted earlier, the selection of the L and C components determines the frequency to which the filter is tuned (see Equation 1 on page 14), but you must be careful because the upstream circuit inductance is present, and the combination of upstream inductance interacting with the filter inductance creates a secondary filter response point, as described by the following equation:
hr = 1 ÷ 2ϖ√[(Lupstream + Lfilter) × C]
Although this secondary response can further serve to reduce the filter response to upstream harmonics, it can also create an unwanted resonant response characteristic.
In Part 2 of this article, we'll discuss filter design and various system parameters that affect filter performance.
Shaughnessy is vice president, PowerCET Corp., Santa Clara, Calif. He can be reached at [email protected].