Just because harmonics is becoming a more prevalent problem, that doesn't mean the subject is getting any easier to understand
Harmonics are AC voltages and currents with frequencies that are integer multiples of the fundamental frequency. On a 60-Hz system, this could include 2nd order harmonics (120 Hz), 3rd order harmonics (180 Hz), 4th order harmonics (240 Hz), and so on. Normally, only odd-order harmonics (3rd, 5th, 7th, 9th) occur on a 3-phase power system. If you observe even-order harmonics on a 3-phase system, you more than likely have a defective rectifier in your system.
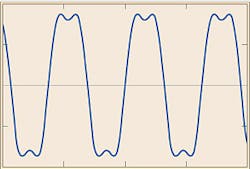
If you connect an oscilloscope to a 120V receptacle, the image on the screen usually isn't a perfect sine wave. It may be very close, but it will likely be different in one of several ways. It might be slightly flattened or dimpled as the magnitude approaches its positive and negative maximum values (Fig. 1). Or perhaps the sine wave is narrowed near the extreme values, giving the waveform a peaky appearance (Fig. 2 below). More than likely, random deviations from the perfect sinusoid occur at specific locations on the sine wave during every cycle (Fig. 3 below).
The flattened and dimpled sinusoid in Fig. 1 has the mathematical equation, y=sin (x)+0.25 sin (3x). This means a 60-Hz sinusoid (the fundamental frequency) added to a second sinusoid with a frequency three times greater than the fundamental (180 Hz) and an amplitude ¼ (0.25 times) of the fundamental frequency produces a waveform similar to the first part of Fig. 1. The 180-Hz sinusoid is called the third harmonic, since its frequency is three times that of the fundamental frequency.
Similarly, the peaky sinusoid in Fig. 2 has the mathematical equation, y=sin (x) -0.25 sin (3x). This waveform has the same composition as the first waveform, except the third harmonic component is out of phase with the fundamental frequency, as indicated by the negative sign preceding the “0.25 sin (3x)” term. This subtle mathematical difference produces a very different appearance in the waveform.
The waveform in Fig. 3 contains several other harmonics in addition to the third harmonic. Some are in phase with the fundamental frequency and others out of phase. As the harmonic spectrum becomes richer in harmonics, the waveform takes on a more complex appearance, indicating more deviation from the ideal sinusoid. A rich harmonic spectrum may completely obscure the fundamental frequency sinusoid, making a sine wave unrecognizable.
Decomposing a distorted waveform into its harmonic components is considerably more difficult. This process requires Fourier analysis, which involves a fair amount of calculus. However, electronic equipment has been developed to perform this analysis on a real-time basis. One manufacturer offers a 3-phase power analyzer that can digitally capture 3-phase waveforms and perform a host of analysis functions, including Fourier analysis, to determine harmonic content. Another manufacturer offers similar capabilities for single-phase applications. Easy-to-use analyzers like these can help detect and diagnose harmonic-related problems on most power systems.
What causes harmonics? If harmonic voltages aren't generated intentionally, where do they come from? One common source of harmonics is iron core devices like transformers. The magnetic characteristics of iron are almost linear over a certain range of flux density, but quickly saturate as the flux density increases. This nonlinear magnetic characteristic is described by a hysteresis curve. Because of the nonlinear hysteresis curve, the excitation current waveform isn't sinusoidal. A Fourier analysis of the excitation current waveform reveals a significant third harmonic component, making it similar to the waveform shown in Fig. 2.Core iron isn't the only source of harmonics. Generators themselves produce some 5th harmonic voltages due to magnetic flux distortions that occur near the stator slots and nonsinusoidal flux distribution across the air gap. Other producers of harmonics include nonlinear loads like rectifiers, inverters, adjustable-speed motor drives, welders, arc furnaces, voltage controllers, and frequency converters.
Semiconductor switching devices produce significant harmonic voltages as they abruptly chop voltage waveforms during their transition between conducting and cutoff states. Inverter circuits are notorious for producing harmonics, and are in widespread use today. An adjustable-speed motor drive is one application that makes use of inverter circuits, often using pulse width modulation (PWM) synthesis to produce the AC output voltage. Various synthesis methods produce different harmonic spectra. Regardless of the method used to produce an AC output voltage from a DC input voltage, harmonics will be present on both sides of the inverter and must often be mitigated.
Effects of harmonics. Besides distorting the shape of the voltage and current sinusoids, what other effects do harmonics cause? Since harmonic voltages produce harmonic currents with frequencies considerably higher than the power system fundamental frequency, these currents encounter much higher impedances as they propagate through the power system than does the fundamental frequency current. This is due to “skin effect,” which is the tendency for higher frequency currents to flow near the surface of the conductor. Since little of the high-frequency current penetrates far beneath the surface of the conductor, less cross-sectional area is used by the current. As the effective cross section of the conductor is reduced, the effective resistance of the conductor is increased. This is expressed in the following equation:where R is the resistance of the conductor, ρ is the resistivity of the conductor material, L is the length of the conductor, and A is the cross-sectional area of the conductor. The higher resistance encountered by the harmonic currents will produce a significant heating of the conductor, since heat produced — or power lost — in a conductor is I2R, where I is the current flowing through the conductor.
This increased heating effect is often noticed in two particular parts of the power system: neutral conductors and transformer windings. Harmonics with orders that are odd multiples of the number three (3rd, 9th, 15th, and so on) are particularly troublesome, since they behave like zero-sequence currents. These harmonics, called triplen harmonics, are additive due to their zero-sequence-like behavior. They flow in the system neutral and circulate in delta-connected transformer windings, generating excessive conductor heating in their wake.
Reducing the effects of harmonics. Because of the adverse effect of harmonics on power system components, the IEEE developed standard 519-1992 to define recommended practices for harmonic control. This standard also stipulates the maximum allowable harmonic distortion allowed in the voltage and current waveforms on various types of systems.Two approaches are available for mitigating the effects of excessive heating due to harmonics, and a combination of the two approaches is often implemented. One strategy is to reduce the magnitude of the harmonic waveforms, usually by filtering. The other method is to use system components that can handle the harmonics more effectively, such as finely stranded conductors and k-factor transformers.
Harmonic filters can be constructed by adding an inductance (L) in series with a power factor correction capacitor (C). The series L-C circuit can be tuned for a frequency close to that of the troublesome harmonic, which is often the 5th. By tuning the filter in this way, you can attenuate the unwanted harmonic.
Filtering isn't the only means of reducing harmonics. The switching angles of an inverter can be preselected to eliminate some harmonics in the output voltage. This can be a very cost-effective means of reducing inverter-produced harmonics.
Since skin effect is responsible for the increased heating caused by harmonic currents, using conductors with larger surface areas will lessen the heating effects. This can be done by using finely stranded conductors, since the effective surface area of the conductor is the sum of the surface area of each strand.
Specially designed transformers called k-factor transformers are also advantageous when harmonic currents are prevalent. They parallel small conductors in their windings to reduce skin effect and incorporate special core designs to reduce the saturation effects at the higher flux frequencies produced by the harmonics.
You should also increase the size of neutral conductors to better accommodate triplen harmonics. Per the FPN in 210.4(A) and 220.22 of the 2002 NEC, “A 3-phase, 4-wire wye-connected power system used to supply power to nonlinear loads may necessitate that the power system design allow for the possibility of high harmonic neutral currents.” And per 310.15(B)(4)(c), “On a 4-wire, 3-phase wye circuit where the major portion of the load consists of nonlinear loads, harmonic currents are present on the neutral conductor: the neutral shall therefore be considered a current-carrying conductor.” It's important to note that the duct bank ampacity tables in B.310.5 through B.310.7 are designed for a maximum harmonic loading on the neutral conductor of 50% of the phase currents.
Harmonics will undoubtedly continue to become more of a concern as more equipment that produces them is added to electrical systems. But if adequately considered during the initial design of the system, harmonics can be managed and their detrimental effects avoided.
Fehr is an independent engineering consultant located in Clearwater, Fla.